Here’s the actual problem my student Dan Thornton spent most of his semester trying to understand and prove. It’s pretty crazy.
The problem: Automaton Identification from Given Data. We abbreviate it “AutID”. G&J call this problem “Minimum Inferred Finite State Automaton” and it is problem AL8 in the appendix.
The description: Given a finite set of data, is it possible to build a deterministic finite automata of ![]() or fewer states that agrees with the data?
or fewer states that agrees with the data?
Prior to giving a description of this problem we must introduce a few concepts and definitions. We want to construct a finite automation, this means a Mealy model deterministic finite automata. Formally a 6-tuple of the form
![]()
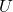 is the input alphabet, where
is the input alphabet, where  is an individual character, and
is an individual character, and 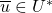 is a string.
is a string.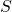 the set of states in the model.
the set of states in the model. 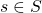 refers to an individual state.
refers to an individual state. 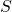 may equivalently be referred to as the set
may equivalently be referred to as the set ![Rendered by QuickLaTeX.com S_{[s]}](https://npcomplete.owu.edu/wp-content/ql-cache/quicklatex.com-b8150e1cdd7a6d8b6757709c274c72aa_l3.png) of all equivalence classes
of all equivalence classes ![Rendered by QuickLaTeX.com [s]_{\overline{u}}](https://npcomplete.owu.edu/wp-content/ql-cache/quicklatex.com-92de2112a12b2d8a768786943b94f7f2_l3.png) , with representative element
, with representative element 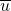 . Each equivalence class is a set of all input strings
. Each equivalence class is a set of all input strings 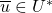 such that
such that 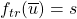 .
.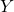 the output alphabet, where
the output alphabet, where 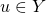 is a individual character,
is a individual character, 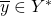 .
.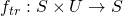 is the transition function.
is the transition function.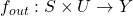 is the output function.
is the output function.
- Both of the above functions may be extended in a natural way so that
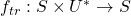 and
and 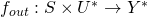 respectively. Usage should be clear from context.
respectively. Usage should be clear from context.
- Both of the above functions may be extended in a natural way so that
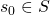 is the start state.
is the start state.
We use the standard convention that ![]() refers to the empty string of no characters.
refers to the empty string of no characters.
A black box is similar to an ‘unknown’ Mealy model with the restriction that for any input string ![]() we only get to see the last output character
we only get to see the last output character ![]() returns. Consider the following black box. With input alphabet
returns. Consider the following black box. With input alphabet ![]() and output alphabet
and output alphabet ![]() .
.
So if the above known black box was passed the string ![]() the complete Mealy model’s output function would give us back the string
the complete Mealy model’s output function would give us back the string ![]() where as the black box would only give us back
where as the black box would only give us back ![]() .
.
Now our above finite automata is constructed using data we are given, here we assume that data is a set ![]() of finite pairs of the form
of finite pairs of the form ![]() .
.
![]() such that
such that ![]() is not empty and
is not empty and ![]() for
for ![]() .
.
An automata ![]() agrees with data
agrees with data ![]() if for every datum
if for every datum ![]() , the final output character of
, the final output character of ![]() .
.
Formal Problem Statement:
Given input alphabet ![]() , output alphabet
, output alphabet ![]() and data, a set
and data, a set ![]() of finite pairs determine if an automation of
of finite pairs determine if an automation of ![]() or fewer states exists that agrees with D.
or fewer states exists that agrees with D.
The Reduction:
The reduction by Gold uses Monotone EQ SAT. So we are given a conjunction of clauses ![]() where each clause in
where each clause in ![]() contains all negated or non-negated variables
contains all negated or non-negated variables ![]() and the number of clauses and variables is equal, is there an assignment of the variables so that
and the number of clauses and variables is equal, is there an assignment of the variables so that ![]() is satisfied? Our instance will have
is satisfied? Our instance will have ![]() clauses and variables. Clauses will be numbered
clauses and variables. Clauses will be numbered ![]() and variables will be numbered
and variables will be numbered ![]() .
.
In the above instance of Monotone EQ SAT we do not have a variable ![]() . Indices in our reduction will sometimes force us to reference
. Indices in our reduction will sometimes force us to reference ![]() . We may think of this variable as simply a place holder for
. We may think of this variable as simply a place holder for ![]() .
.
The idea behind this reduction is that we may encode our instance of Monotone EQ SAT into data ![]() in such a way that if we can create a finite automata from our encoding, then we can use it to obtain a solution to the instance of Monotone EQ SAT if one exists.
in such a way that if we can create a finite automata from our encoding, then we can use it to obtain a solution to the instance of Monotone EQ SAT if one exists.
Aut ID construction:
Here we transform our instance of Monotone EQ SAT into data ![]() , to do so we need the following functions:
, to do so we need the following functions:
![]()
![]()
The bounds on the above functions are a result of our numbering of clauses ![]() and
and ![]() . Note that
. Note that ![]() is really a placeholder for
is really a placeholder for ![]() .
.
Given a propositional formula ![]() which is an instance of MonotoneEQ SAT we encode it by the following:
which is an instance of MonotoneEQ SAT we encode it by the following:
- The variables of
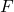 are encoded by the set
are encoded by the set
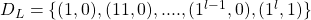 . Here each
. Here each 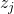 corresponds to the element
corresponds to the element 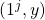 where
where 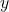 is an element of the output alphabet,
is an element of the output alphabet, 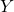 .
. - We encode the clauses
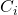 of
of 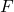 by
by  for
for 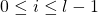 ,
, 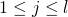 . Here each clause has a \textit{identifying tag},
. Here each clause has a \textit{identifying tag}, 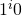 , then the
, then the 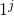 term is used specify a particular variable,
term is used specify a particular variable, 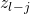 . Importantly, datum in this set for which
. Importantly, datum in this set for which  returns
returns 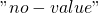 are thrown out.
are thrown out.
- Each clause encoding
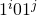 will be
will be  only if clause
only if clause 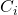 does not contain variable
does not contain variable 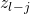 , otherwise
, otherwise  will be
will be 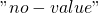 and is thrown out.
and is thrown out.
- Each clause encoding
- We then encode whether each clause is a set of all negated, or non-negated variables.
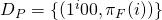 for
for 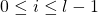 .
.
As a simple example we convert to data the following formula
![]()
So after our conversion we get:
Aut ID instance
Now we create an instance of ![]() by letting
by letting ![]() be the number of variables
be the number of variables ![]() and
and ![]() .
.
True{Aut ID} ![]() True{Monotone EQ SAT}
True{Monotone EQ SAT}
Now we may assume that we have a finite automata ![]() that agrees with the data in
that agrees with the data in ![]() . Now we may use the transition function
. Now we may use the transition function ![]() and the output function
and the output function ![]() to deduce properties of
to deduce properties of ![]() .
.
Let ![]() be
be ![]() , this is the state that
, this is the state that ![]() is in after receiving the input string
is in after receiving the input string ![]() .
.
A splitting string of ![]() and
and ![]() is a string
is a string ![]() so that
so that ![]() differs from
differs from ![]() . If such a string exists then obviously
. If such a string exists then obviously ![]() . If two states do not have splitting string then they are equivalent
. If two states do not have splitting string then they are equivalent
We define variable states as follows, a variable ![]() has the encoding
has the encoding ![]() , so
, so ![]() is
is ![]() ‘s variable state, we will sometimes refer to this as
‘s variable state, we will sometimes refer to this as ![]() .
.
Clause states are defined in a manner similar to variable states. A clause ![]() corresponds to the identifying tag in the encoding of
corresponds to the identifying tag in the encoding of ![]() or
or ![]() , so
, so ![]() is
is ![]() ‘s clause state, we will sometimes refer to this as
‘s clause state, we will sometimes refer to this as ![]() .
.
In the following theorems we shall talk of assignment of clause state ![]() to variable state
to variable state ![]() , this means that these two states are equivalent or alternatively that there does not exist a splitting string of
, this means that these two states are equivalent or alternatively that there does not exist a splitting string of ![]() and
and ![]() .
.
Theorem 1:All variable states are different.
Proof. We assume two variable states are equal ![]() and
and ![]() where
where ![]() . Then in the encoding of
. Then in the encoding of ![]() we get
we get ![]() and
and ![]() respectively. But now we may define the splitting string
respectively. But now we may define the splitting string ![]() . The final element of
. The final element of ![]() is defined in our data
is defined in our data ![]() as
as ![]() . By a similar argument we get
. By a similar argument we get ![]() where
where ![]() , in our data this corresponds to one of the entries
, in our data this corresponds to one of the entries ![]() . Our automata
. Our automata ![]() must agree with the data, so as a result these states
must agree with the data, so as a result these states ![]() and
and ![]() cannot be equal. Thus we have reached a contradiction.
cannot be equal. Thus we have reached a contradiction. ![]()
Theorem 2: Each state in our finite automata ![]() corresponds to exactly one variable.
corresponds to exactly one variable.
Proof. The above follows by the pigeonhole principle, as ![]() has exactly
has exactly ![]() states, there are
states, there are ![]() variable states, and by Theorem 1, no two variable states are equal.
variable states, and by Theorem 1, no two variable states are equal. ![]()
By the above we may talk about the states of ![]() in term of the variable each state corresponds to.
in term of the variable each state corresponds to.
Theorem 3: If clause state ![]() and variable state
and variable state ![]() are equal, Then clause
are equal, Then clause ![]() contains variable
contains variable ![]() .
.
Proof. Assume ![]() =
= ![]() and
and ![]() is not contained in
is not contained in ![]() . Once again we have the following encodings
. Once again we have the following encodings ![]() and
and ![]() that were defined in our construction of
that were defined in our construction of ![]() . Now we may define a splitting string
. Now we may define a splitting string ![]() . When we append the splitting string to each of the above encodings we get
. When we append the splitting string to each of the above encodings we get ![]() and
and ![]() . Both of these strings are defined in our data
. Both of these strings are defined in our data ![]() as
as ![]() and
and ![]() respectively, and by our assumption that
respectively, and by our assumption that ![]() is not contained in
is not contained in ![]() ,
, ![]() So by the a conclusion identical to that of Theorem 1,
So by the a conclusion identical to that of Theorem 1, ![]() and our theorem follows.
and our theorem follows. ![]()
Theorem 4:No two clause states ![]() and
and ![]() may be equal if they are of opposite composition, meaning one has all negated variables, and the other all un-negated variables.
may be equal if they are of opposite composition, meaning one has all negated variables, and the other all un-negated variables.
Proof. Once again we proceed by contradiction, assume ![]() and that
and that ![]() ,
, ![]() are of opposite composition. Once again we get each clauses encoding
are of opposite composition. Once again we get each clauses encoding ![]() and
and ![]() respectively. Now we may define the splitting string
respectively. Now we may define the splitting string ![]() . Looking at the final character output by
. Looking at the final character output by ![]() we see
we see ![]() and
and ![]() . It follows that
. It follows that ![]() as
as ![]() and
and ![]() are of opposite composition. Thus we arrive at a contradiction.
are of opposite composition. Thus we arrive at a contradiction.![]()
Theorem 5: ![]() gives us a solution to
gives us a solution to ![]() our instance of
our instance of ![]() .
.
Proof. By Theorem 2 every clause state must be assigned to a single variable state, notice this does not mean only a single assignment is possible but just the given automata ![]() will give a single assignment. By Theorem 1 no clause may be assigned more than one variable state. By Theorem 3 a clause may be assigned to a variable state only if the clause contains the variable. So we see that our automata
will give a single assignment. By Theorem 1 no clause may be assigned more than one variable state. By Theorem 3 a clause may be assigned to a variable state only if the clause contains the variable. So we see that our automata ![]() assigns clauses to the variables that could potentially satisfy them. Furthermore by Theorem 4, each variable state is given clauses of only one composition, so there is an obvious variable assignment to satisfy all of the clause states equal to a given variable state.
assigns clauses to the variables that could potentially satisfy them. Furthermore by Theorem 4, each variable state is given clauses of only one composition, so there is an obvious variable assignment to satisfy all of the clause states equal to a given variable state. ![]()
True{Monotone EQ SAT} ![]() True{Aut ID}
True{Aut ID}
Now we assume there exists a mapping ![]() that satisfies our instance of Monotone EQ SAT,
that satisfies our instance of Monotone EQ SAT, ![]() with
with ![]() clauses and variables. From this mapping we build a finite automata
clauses and variables. From this mapping we build a finite automata ![]() that agrees with all data
that agrees with all data ![]() .
.
We let ![]() ‘s 0-transitions refer to transitions on input 0. Similarly 1-transitions refer to the transitions on input 1.
‘s 0-transitions refer to transitions on input 0. Similarly 1-transitions refer to the transitions on input 1.
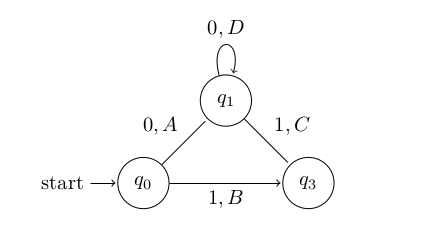
So first we define all 1-transitions as given in the following example automata, obviously such transitions will satisfy all data in ![]() .
.
Now for ![]() to satisfy all of the data in
to satisfy all of the data in ![]() we notice by Theorems 3 and 4 above mapping each clause to the variable state that satisfies it seems like a natural thing to do. We may determine this mapping of clauses to the variables that satisfy them from our truth assignment that satisfies
we notice by Theorems 3 and 4 above mapping each clause to the variable state that satisfies it seems like a natural thing to do. We may determine this mapping of clauses to the variables that satisfy them from our truth assignment that satisfies ![]() ,
, ![]() .
.
Such mappings may be added to ![]() by noticing the following, as soon as we encounter a 0 in input we must transition to a clause state. So we may define a 0-transition for every state in
by noticing the following, as soon as we encounter a 0 in input we must transition to a clause state. So we may define a 0-transition for every state in ![]() that maps the clause state
that maps the clause state ![]() (which recall corresponds to the string
(which recall corresponds to the string ![]() ) to the variable state
) to the variable state ![]() that satisfies it. When adding 0-transitions we must be careful as if clause
that satisfies it. When adding 0-transitions we must be careful as if clause ![]() is satisfied by variable
is satisfied by variable ![]() then in order to agree with the data in
then in order to agree with the data in ![]() we really should map
we really should map ![]() to variable
to variable ![]() due to the definition of
due to the definition of ![]() .
.
Now we must assign an output to each of these 0-transitions. To do so we iterate through every clause state ![]() by sequentially considering
by sequentially considering ![]() . At each clause state we assign the output of the 0-transition starting at this state to be
. At each clause state we assign the output of the 0-transition starting at this state to be ![]() . Notice that if two clause states
. Notice that if two clause states ![]() and
and ![]() are identical, then they must both be satisfied by the same variable and so therefore
are identical, then they must both be satisfied by the same variable and so therefore ![]() .
.
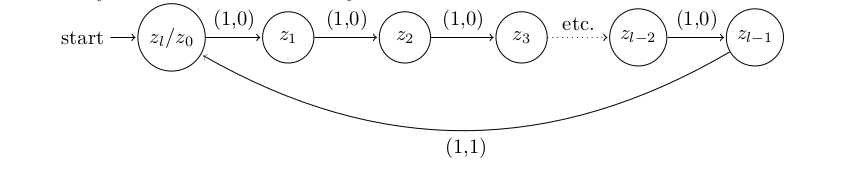
Below is an example of adding such a 0-transition and assigning its output:
The Blue edge is the zero transition added that maps the clause state ![]() ,which corresponds to the string
,which corresponds to the string ![]() , to the variable state
, to the variable state ![]() . Semantically this means that the variable
. Semantically this means that the variable ![]() satisfies this clause.
satisfies this clause.
The Red edge shows us adding the output value of a 0-transition, as from the above blue edge we know ![]() corresponds to the clause
corresponds to the clause ![]() ‘s clause state, so to the zero transition from this clause state will have output
‘s clause state, so to the zero transition from this clause state will have output ![]() .
.
Theorem 6: Our constructed automata ![]() agrees with all
agrees with all ![]()
Proof. This is quite obvious from our example of ![]() in Figure 1. We have defined all the
in Figure 1. We have defined all the ![]() transitions, and notice that the final output of all
transitions, and notice that the final output of all ![]() if
if ![]() and
and ![]() this exactly matches our data
this exactly matches our data ![]() .
. ![]()
Theorem 7: Our constructed automata ![]() agrees with all
agrees with all ![]()
Proof:Here we consider the final character output of ![]() for all encodings of fixed clause
for all encodings of fixed clause ![]() , these encodings are precisely the
, these encodings are precisely the ![]() for
for ![]() . Now with the addition of the
. Now with the addition of the ![]() -transitions, we transition into
-transitions, we transition into ![]() , and from this state we only worry about
, and from this state we only worry about ![]() -transitions. Recall that in our definition of
-transitions. Recall that in our definition of ![]() , all datum are of the form
, all datum are of the form ![]() . In
. In ![]() there is a single
there is a single ![]() -transition that outputs the non-zero value
-transition that outputs the non-zero value ![]() . To agree with
. To agree with ![]() it is sufficient to make sure that this non-zero output occurs for an input string not in
it is sufficient to make sure that this non-zero output occurs for an input string not in ![]() .
.
From our assignment of ![]() -transitions defined above this singular
-transitions defined above this singular ![]() -transition that outputs
-transition that outputs ![]() will occur for the variable
will occur for the variable ![]() that satisfies
that satisfies ![]() , so then
, so then ![]() must be in
must be in ![]() . So
. So ![]() will give the datum (
will give the datum (![]() ,1). Now notice the corresponding entry in
,1). Now notice the corresponding entry in ![]() as defined by
as defined by ![]() would be (
would be (![]() ,
,![]() ), but all of these datum were removed from
), but all of these datum were removed from ![]() . So the sufficiency condition above is satisfied.
. So the sufficiency condition above is satisfied. ![]()
Theorem 8 :Our constructed automata ![]() agrees with all
agrees with all ![]()
Proof: The manner in which we defined our 0 transitions explicitly involved a ![]() term, so for any clause state
term, so for any clause state ![]() , now a 0 transition as defined above will output
, now a 0 transition as defined above will output ![]() which will obviously agree with
which will obviously agree with ![]()
By Theorem 6, Theorem 7, and Theorem 8. We conclude that ![]() will agree with data
will agree with data ![]()
(Back to me)
Difficulty: 9. This is basically the reduction in the Gold paper, though Dan reorganized it a bunch to make it fit our format, and be more understandable. But the formatting of the various variables, the fact that you need to define the IF function on variable k to talk about zl-k instead of zk, and the three different kinds of test data all make this super hard to understand. In some ways, I wonder if this should be a 10, given the work we did on it. But I’m saving the 10’s for things that are truly impenetrable, and we did manage to figure this one out.
Hi Prof.,
I had a query related to another problem [AL2] “Two way Finite State Automaton Non-Emptiness” in G&J (I think you haven’t covered it yet.
Essentially, it mentions that determining if a 2Way FSA on a Single Alphabet is empty is NP Complete.
I am confused, about this one. Why can’t we test strings on 1 of all lengths one by one till we find if one is accepted or determine the automata is Empty. Unlike in a Binary Alphabet we don’t have exponential number of possibilities of input strings. And we would have to test strings that are polynomial in size w.r.t. to the size of the given Automata.
So how is this problem NPComplete?
** The automaton is Non-Deterministic (just to clarify).
Well, I haven’t got there, so I don’t know the problem too well. But the way the comments read, I’d say that this _specific_ problem is NP-Complete when sigma is 2, but not when it’s 1. They say that the _general_ problem is NP-Complete when sigma is 1.
So the question is what are they generalizing in the “general” problem? I’m not sure. My guess is that they’re generalizing from “two-way” to “any-way”. So instead of mapping to Qx{-1,0,1}, you can map into QxZ.
But I’m not sure. Have you read the Galil paper that they reference?
No Prof. Unfortunately not.
1. “INSTANCE: A two-way nondeterministic finite state automaton A =(……”
2. “Comment: PSPACE-complete, even if |l|=2 and A is deterministic. If |l| = l the general problem is NP-complete [Galil, 1976]. ”
By general I suppose they mean the nondeterministic variant of the problem. They needed to specify it as in the previous line for a 2 alphabet version they mentioned that ‘even in deterministic case’ its PSPACE complete.
Maybe. I just downloaded the Galil paper and the way they phrase it is “The nonemptiness problem for two way nondeterministic finite automaton languages over one symbol is in NP (in fact it is complete in NP)”
It’s weird that G&J used “general” to mean “nondeterministic” though…
Thank you Prof. (I didn’t have access to the journal so couldn’t recheck myself). Yes its weird/unclear, took me some time too to get what they might have meant. 🙂
I await your post on this as its still unclear why it should be NPComplete. On the face of it, doesn’t seem like it.
Perhaps because of the exponential number of ways the head can move back and forth.
Yeah, the proof starts by saying that if you have a 2-way NFA with N states, there’s an equivalent nfa with n^(n+1) states. So that’s probably where you get the exponential time from.
Hi Prof.
I had some more doubts on yesterday’s discussion. As you explained “2-way NFA with N states, there’s an equivalent nfa with n^(n+1) states. So that’s probably where you get the exponential time from.”
I am clear about that, but if I understand correctly the size of the string accepted by the 2Way NFA would still be (in the worst case) polynomial to number of states in the 2Way NFA. Cause if the smallest string accepted was exponential in size, the verification will also take exponential time and thus the problem by definition would not be NP Complete ? Or is there a mistake somewhere in understanding.
Since I don’t have the access to paper I am not sure if an exponential sized accepting sting can be verified in polynomial time (or have the mentioned about input size verification in general)
Or instead of using an input string as witness they are using some other method to verify the non emptiness that can be done in polynomial time ?
It looks like they’re using it to build a nondeterministic algorithm. It’s not a regular reduction like we normally do. They use it to show that if the 2-way NFA accepts a string, it needs to be bounded by n^(n+1) in size (and thus the tape is bounded as well). Then they count configurations of states and heads and show how to build an algorithm to solve the problem. The paper was written before the “normal” ways of doing reductions really came about, and I don’t know a lot about how you prove things directly from the machines.
Thank you Prof.
I too was unable to find this Problem in any of the modern books or any related reduction in our modern form either. Seems a lot complicated as compared to our standardized form too.