Protected: Reynolds Covering for Context-Free Grammars
Enter your password to view comments.
Tag Archives: Clique
Protected: Partially Ordered Knapsack
Enter your password to view comments.
Protected: Conjunctive Boolean Query
Enter your password to view comments.
Protected: K-Closure
Enter your password to view comments.
Protected: Maximum Subgraph Matching
Enter your password to view comments.
Protected: Induced Subgraph with Property π, Induced Connected Subgraph with Property π
Enter your password to view comments.
Protected: Balanced Complete Bipartite Subgraph
Enter your password to view comments.
Protected: Largest Common Subgraph
Enter your password to view comments.
Clique
G&J don’t even bother to prove Clique is NP-Complete, just stating that it (and Independent Set) is a “different version” of Vertex Cover. But the problem comes up often enough that it’s worth seeing in its own right.
The problem: Clique (I’ve also seen “Max Clique” or “Clique Decision Problem” (CDP))
The definition: Given a graph G=(V,E), and a positive integer J (G&J say J needs to be ≤ |V|, but I think you can allow large values and make your algorithm just say “no”). Does J contain a subset V’ of V, or size at least J, where every two vertices in V’ are joined by an edge in E?
Example: Using the graph we had for VC:
{a,c,d} form a clique of size 3. There are no cliques of size 4, but if we add the edge (d,g) and the edge (c,h), then the vertices {c,d,g,h} would be one.
The reduction: From Vertex Cover. Given a graph G and an integer K that is an instance of Vertex Cover, take the complement of G (the complement of a graph has the same vertices, but the set of edges is “inverted”: (u,v) is an edge in the complement if and only if it was not an edge in G), and use it as your instance of CDP. Set J= |V|-K
Difficulty: 3. It’s not a 2 because it takes a little thought to see why the complement works.
Note: As stated perviously, I teach this course using the Cormen book, so in class I show Clique is NP-Hard by reducing from 3SAT. It’s a good example of a hard reduction, probably about as hard as the VC reduction G&J do.
Vertex Cover
Back to the “core 6” with a classic problem from graph theory. There are lots of similar graph problems, so it’s important to keep them all straight.
The Problem: Vertex Cover (VC)
The Definition: Given a graph G=(V,E) and a positive integer K (G&J say that K ≤ |V|, but really, if it’s bigger than that, you can have the algorithm just return “yes”). Can I find a subset V’ of V, |V’| ≤ K, where every edge in E has at least one endpoint in V’?
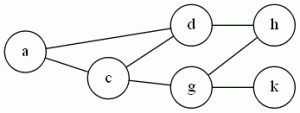
Example: Hmm, I wonder if I can insert an image..
Ok, looks like that worked. In the graph above, {c,d,g} is a vertex cover, because all edges have at least one endpoint in the set {c,d,g}. As we’ll see later, a graph containing a clique (complete subgraph) of N nodes will need at have at least N-1 of those vertices in the cover.
The reduction: From 3SAT. G&J on pages 54-56 show the construction. We build a graph with vertices for all variables and their negation, with an edge between them. We have three variables for each clause, connected in a triangle. (The three variables correspond to “positions” in the clause). Then there is an edge from each vertex corresponding to a literal (or its negation) to its corresponding “clause vertex”- the vertex that holds the position of that variable in the original formula. K= # of variables + 2* number of clauses.
Difficulty: 7 , assuming it’s the first time a student has seen this sort of reduction. If they have seen something similar (for example, a reduction from 3SAT to Clique), then maybe a 5. The ideas are pretty similar.
Note: I teach my algorithms class using the Cormen book. In it, they reduce 3SAT to Clique, proving Clique is NP-Complete, and then reduce Clique to VC. This works in the exact same way as the reduction from VC to Clique that I’ll be doing here next.